Online Course
NRSG 795: BIOSTATISTICS FOR EVIDENCE-BASED PRACTICE
Module 3: Variation and the Normal Distribution
Estimating Population Values from Samples
In this section we learn to estimate a population value from the sample (click for more information).
Confidence Intervals
A point estimation involves calculating a single statistic for estimating the parameter. However, point estimates do not give you a context for interpreting their accuracy. In order to overcome this issue, an interval estimation can be performed which gives a range of values that has a high probability of containing the true population value. This is commonly referred to as a confidence interval. Within EBP context, CI is used as means for appraising the evidence, i.e. the precision of the estimate of effects.
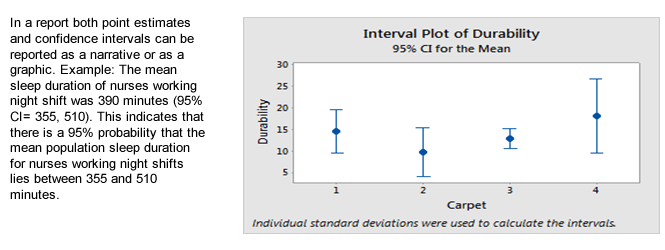
The 95% confidence interval is commonly used. It means there are 5 chances in 100 of making an error (this can also be set at 99% or 1 chance in 100 of making an error).
Confidence intervals can be computed around any point estimate such as a mean, percentage, or risk index. For example the 95% CI around a sample mean [point estimate] can be calculated using the formula CI 95% = (Mean ± 1.96 * SD) but this should not be confused with calculating the confidence interval for a population value [parameter estimate]. The problem with point estimates is they are simply descriptive stats and offer no context for interpreting their accuracy. So instead we prefer to estimate a range of values that has a high probability of containing the population value. In order to do that we first must estimate the standard error of the mean.
Standard error of the mean
Standard Error is essentially a standard deviation of a population. A standard error of the mean (SEM) is the standard deviation (SD) of a sampling distribution of the mean. It represents the error expected when the mean from a small sample is used to calculate the SD of the population mean. The term error is used to show that there is sampling error or that the sample means contain some degree of error in estimating the population mean. The term ‘standard’ means that it is a measure of the average amount of error for all possible sample means. Since most sampling distributions are approximately normal, the SEM can be used to estimate the probability of getting a sample mean in a particular range.
SEM= SD/√N
A 95% confidence interval is computed from the mean plus or minus two SEMs
Remember that 95% of the values in a normal distribution lie within + 2 SDs of the mean; so the probability is .95 that a particular mean will be between the two values at +2SD and -2SD. With information about the SEM, sample means can be interpreted relative to the population mean. When the SEM is smaller, there is greater confidence in the estimates of the population value.
CI 95% = (Mean ± 1.96 *SEM) CI 99% = ( Mean ± 2.58 *SEM)
Since the formula for SEM contains the square root of the population (square root of N) in the denominator, a larger sample size increases the probability that the sample mean will be close to the population mean. In order to decrease the SEM, researchers can increase the sample size. The formula for SEM also has standard deviation (SD) as the numerator, so the smaller the SD (or the greater the homogeneity) of the sample, the smaller is the SEM.
It is easy to be confused about the difference between the standard deviation (SD) and the standard error of the mean (SEM). Here are the key differences:
- The SD quantifies scatter — how much the values vary from one another.
- The SEM quantifies how precisely you know the true mean of the population. It takes into account both the value of the SD and the sample size.
Required Videos
- Central Limit Theorem video (9:48)
- Understanding Confidence Intervals (4:02)
- Calculating the Confidence Interval for a Mean (5:28)
- NOT REQUIRED: article with more information
Learning Activity
This website is maintained by the University of Maryland School of Nursing (UMSON) Office of Learning Technologies. The UMSON logo and all other contents of this website are the sole property of UMSON and may not be used for any purpose without prior written consent. Links to other websites do not constitute or imply an endorsement of those sites, their content, or their products and services. Please send comments, corrections, and link improvements to nrsonline@umaryland.edu.